For anyone who has taking an entry level calculus class, the fundamental theorem of calculus is a cornerstone of advanced mathematics that allows us to find areas under curves in the xy plane. More importantly, this theorem can be applied to many real world problems in the fields of physics and engineering. In other words, it is a backbone of the calculations that support the world all around us. Wikipedia defines the theorem as follows:

“The fundamental theorem of calculus is a theorem that links the concept of differentiating a function (calculating its slopes, or rate of change at each time) with the concept of integrating a function (calculating the area under its graph, or the cumulative effect of small contributions). The two operations are inverses of each other apart from a constant value which depends on where one starts to compute area.

The first part of the theorem, the first fundamental theorem of calculus, states that for a function f , an antiderivative or indefinite integral F may be obtained as the integral of f over an interval with a variable upper bound. This implies the existence of antiderivatives for continuous functions.

Conversely, the second part of the theorem, the second fundamental theorem of calculus, states that the integral of a function f over a fixed interval is equal to the change of any antiderivative F between the ends of the interval. This greatly simplifies the calculation of a definite integral provided an antiderivative can be found by symbolic integration, thus avoiding numerical integration.”

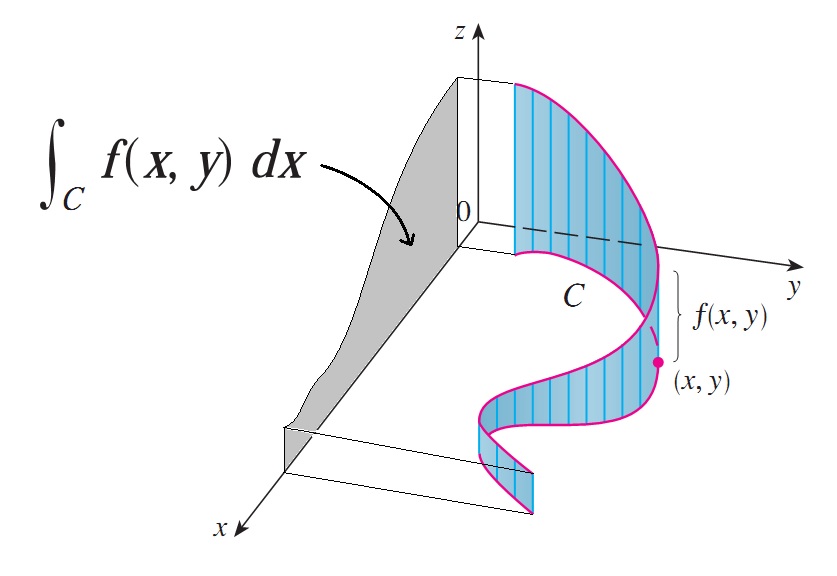
However, even though the theorem is incredibly applicable to real world problems bound by two dimensions, we live in three dimensional world. Meaning that mathematicians often have to face problems of integrating a vector field over a region in three dimensional space as opposed to integrating an area under a curve in two dimensional space. For problems like these, we can use the fundamental theorem of multivariable calculus. Which says that for a conservative vector field in the real plane, the work done between two points is independent of path and is equal to the anti-gradient function of the field evaluated at the terminal point minus the same function evaluated at the initial point. This theorem is very powerful because it allows mathematicians to evaluate the work between two points in a vector field without actually knowing that path over which a particle may travel that the work is done on.